4.1.1 Unit Locations and the Pattern of an Activity

So far, we have considered only the locational preferences and decisions of the individual unit. We now move to a different level of inquiry, in which attention is focused on the patterns in which similar units array themselves.

We shall refer to an activity as a category of closely similar location units.¹ In manufacturing, one speaks of an "industry"—such as flour milling or job printing; in trade or services, of a "line" of business. We shall extend the term "activities" to cover analogous groupings, such as residential units of a particular class, types of public facilities with a particular function, and so on. Thus in a given city, the fire department is an activity, with a spatial pattern comprising the locations of fire houses.

Location patterns can take various forms, as can be seen when one sets out to map the locations of different activities. The pattern of the copper smelting industry could be shown as a small number of dots, each representing one smelter. Fashion garment factories are found mainly in tight clusters (such as in midtown Manhattan), each of which contains many firms. Automobile dealers in urban areas tend to be concentrated in linear clusters. Particular crops, or types of farming, are often found in continuous zones, which they preempt to the exclusion of any other major activity.

Sometimes the location pattern of an activity is a planned configuration because there is just a single decision unit involved. In a nonsocialized economy, however, this situation is confined essentially to certain types of public facilities (such as schools within a city school system) and to the few lines of private businesses that are controlled by total monopolies. More characteristically, the location pattern of an activity is the unplanned outgrowth of the behavior of many location decision units.

4.1.2 Competition and Interdependence

As already noted in Chapter 2, individuals and business firms (particularly new and small firms) must make location decisions in the face of great uncertainty, and they are strongly influenced by personal preferences and constraints not closely related to any calculation of money cost, revenue, or profit. But the location pattern of an activity as a whole cannot be understood simply in terms of the factors governing individual unit locations. Here we have to recognize explicitly the role of competition and other kinds of locational interdependence among units.

First, there is the process of competitive weeding out and survival. Establishment of new locations is only one of the ways in which the locational pattern of an activity is altered. The mortality among new and small firms is high, and establishments are continually being abandoned or converted to other uses. Business locations, whether based on wisdom, profound study, personal whim, or guesswork, have to meet the test of survival.

A good analogy is the scattering of certain types of seeds by the wind. These seeds may be carried for miles before finally coming to rest, and nothing makes them select spots particularly favorable for germination. Some fall in good places and get a quick and vigorous start; others fall in sterile or overcrowded spots and die. Because of the survival of those which happen to be well located, the resulting distribution of such plants from generation to generation follows closely the distribution of favorable growing conditions, So in the location of economic activities, it is not strictly necessary to have both competition and wise business planning in order to have a somewhat rational location pattern emerge: Either alone will work in that direction.²

To be sure, the role of the "invisible hand" in promoting efficient location patterns should not be exaggerated. The survival test may weed out multitudes of small mistakes in location, though at a substantial cost in wasted resources. Big mistakes associated with large-scale operations— for example, in the location of a steel mill or a major transport terminal— are considerably more durable. Not only is the fixed investment greater and the competitive pressure less threatening, but in addition such a facility radically alters its environment. It may attract a variety of complementary activities; and, in any event, it will build up a larger local market, thus partly "justifying itself." The need for informed planning of locations in order to forestall misallocation of resources is obviously greater where large-scale units or complexes are involved. Such major decisions are in fact based on more objective criteria and fuller information than is the typical small-unit location.

Competition among business firms is just one of the manifold ways in which locations depend on one another—a dependence that we conveniently ignored in Chapter 2 when considering just one location unit at a time. Whether they be factories, stores, public facilities, offices, or homes, individual location units are never indifferent to locations of other units of the same kind but can be either repelled or attracted by them. Proximity can be an advantage or a disadvantage, or sometimes both at the same time. Our focus in this chapter is on activity patterns shaped by mutual repulsion among the units, or dispersive forces. We will find that the nature of competition in a spatial context may contribute to these forces but that some aspects of spatial competition may have contervailing effects. Subsequently, in Chapter 5, we will consider the contrasting kinds of patterns in which mutual attraction, or agglomerative forces, dominate.

4.1.3 Some Basic Factors Contributing to Dispersed Patterns

Business firms often go to some pains to select locations where there is no nearby competition; and householders likewise shy away from too much proximity to other households, whether from a desire to avoid high rents or congestion, a desire for privacy, or a dislike for some particular category of neighbors. These are instances of locational repulsion among units of the same specific or general type. But several basic reasons for a dispersed pattern can be identified.

One reason is competition for scarce local inputs, such as land, privacy, quiet, or clean air or water. A high concentration of occupancy makes these local inputs more scarce and more expensive. It also discourages further concentration. The importance of this effect is so great that we shall devote Chapter 6 to exploring it in detail.

Another reason for an activity to have a dispersed pattern is that the activity is output-oriented and its markets are dispersed. Thus an effective demand for convenience goods exists wherever there are people with income; and a closely market-oriented activity, such as drugstores, will have a pattern resembling that of population or consumer income. The individual stores prefer locations apart from one another, because they are selling basically the same items and the customers will tend to patronize the nearest store. The demand for the goods of any one store will be greater where there is little or no nearby competition. As a result of this mutual repulsion, the stores are widely distributed. The degree of dispersion (the closeness of fit to the market pattern) is limited only by the high costs of operating a small store, and the pattern thus represents an economic compromise between the factors of market access and scale economy.

Where scale economies call for still further restriction on the number of separate units that can survive, we find individual units selecting not just neighborhoods but cities or regions on a similar basis of avoidance of proximity: They try to find a relatively undersupplied area where the competition is least intense. In both the intracity and the intercity situations, the individual unit has a "market area" within which it has the advantage of better access to the market than its competitors.

Similarly there are activities, oriented to the supply of transferable in puts, that tend to have a dispersed pattern because the pattern of input sources is itself dispersed. Crop-processing activities in agricultural areas are an example. Individual cheese factories, sugar refineries, and the like repel one another in the sense that each can get its inputs more cheaply or easily if it has a "supply area" to itself and is to that extent insulated from competition.

We shall now examine more closely these types of activity patterns involving market areas or supply areas. For brevity’s sake, the discussion will refer basically to market areas, but it should be kept in mind throughout that the same principles apply to supply areas as well.

4.2 MARKET AREAS

4.2.1 Introduction

First, we may note that the importance of keeping a distance from one’s rivals, and the feasibility of carving out a market area, depend on the degree of interchangeability of one’s products with those of the competitors. If the products are not closely standardized, the buyers cannot be relied on to prefer the cheapest nor to patronize the nearest seller. But if the products are standardized, there are likely to be considerable scale economies in producing them, since there is relatively great scope for mechanization and even automation of processes, and the organization and management problems are simpler.

Some economies of large scale refer to the size of the individual establishment or location unit, while others depend primarily on how large the firm or other decision unit is. The economically justifiable size of the individual location unit is constrained by the fact that larger size requires a larger market area and increased transfer costs; but the size of the firm is not under that constraint and may be associated with such substantial savings in costs of management, purchasing, research, advertising, and finance that it is profitable for one firm to operate a number of separate location units. Branch plants are increasingly common in manufacturing and utilities, as are chain stores in retailing. Within the past few decades, multiunit firms have assumed a notably larger role in such activities as hotels and motels, automobile rental, restaurants, theaters, and university education. This trend probably reflects, at least in part, the improvement of communications, data processing, and management techniques, which have widened the scope of economies of large-scale management more than they have affected the scale of individual establishments.

Consequently, one of the important types of market-area patterns is that involving the sales or service areas of different branch units of a single firm—here the relationship among units is obviously different from what it is when the units belong to rival firms.

4.2.2 The Market Area of a Spatial Monopolist

The development of our discussion concerning market-area patterns will be facilitated if we first understand the factors contributing to the market boundary of a spatial monopolist. Whether we choose to think of this monopolist as a branch unit or a single-unit firm with decision-making power is immaterial at present.

The characteristic that distinguishes a firm or a particular location unit as having monopoly power is that when its price is raised, at least some of its customers will remain. No such advantage accrues to the perfect competitor. Its demand curve is such that it has no control over price; any increase in price will cause all of its customers to find alternatives. Most introductory textbooks in economics stress a number of reasons why monopolies can arise (patents, scale economies, etc.), but they neglect the fact that space itself may impart monopoly power. For example, customers in the immediate vicinity of a grocery store are, in a sense, attached to it. Price increases may be tolerated by these customers because switching to an alternative supplier would involve extra time, trouble, and expense. This principle applies equally to many nonbusiness establishments as well. For example, clients of a local free legal or health care service may be willing to tolerate increases in waiting time or other small decreases in the quality of services rendered for much the same reason. The search for alternatives that might exist in other parts of the community is costly.

It is possible to identify the area over which this influence might be exerted by making use of the concept of delivered price introduced in Chapter 2. For ease of presentation, consider initially a unit whose customers are evenly distributed over a linear market; for example, strung along a street or other transfer route. We might think of the seller as charging a uniform f.o.b. price (that is, price at its own location, before transfer) to all buyers, so that each buyer must pay that price plus all expenses associated with transfer to his or her location.

The arrangements by which the buyer pays transfer costs can take several forms. For example, the seller may take responsibility for delivery, and may either move the goods itself or contract with a transfer agency; but in either case it charges the buyer a delivered price that includes all transfer costs. Alternatively, buyers may contract with the transfer agency or move the goods themselves. This last practice is of course common in retail trade, where the buyer takes possession of the product at the seller’s location. For our immediate purposes, it is not necessary to distinguish among these alternatives; in any case we shall assume that delivered price increases with distance, so that the buyer in effect pays all transfer charges.

Under these circumstances, it is particularly easy to identify the market area realized by the seller and to recognize the nature of pricing and output decisions. Let the price at the seller’s location be denoted by p₀ in panel (a) of Figure 4-1. We shall refer to this as the f.o.b. price. Our assumption that the full cost of transfer is reflected in the price that buyers pay implies that a buyer located at some distance from the seller, say d₁, would face a delivered price of p_I, where the amount p₁— p₀represents the transfer cost component. Note that the slope of the delivered price schedule shown in panel (a) is determined by the transfer rate. If we think of distance on the horizontal axis as being measured in miles, then the increment in delivered price associated with the transfer of one unit over one mile is the transfer rate.

In panel (b), the line D represents the demand curve of a typical buyer, and we shall assume that all individuals in the market have identical demand curves. This being so, we can identify the quantity demanded by the buyer located at d₁ as q₁. That is, we recognize that the quantity demanded depends on delivered price. Using panel (c) as an intermediary or mapping device to get us around the corner to panel (d), we can plot the quantity/distance function, which relates the quantity demanded by a buyer to distance from the seller’s location. Thus an individual who is adjacent to the seller will purchase the quantity q₀, and the quantity demanded is zero when the customer is confronted with a delivered price of p₂• For this particular f.o.b. price, p₀, a "natural" market boundary is established at a distance of d₂, where transfer costs have limited the range over which the firm may sell its product or service.

If instead of focusing our attention on a linear market, we allow customers to be distributed over the entire area surrounding this seller, some extensions of this analysis follow immediately. Under this circumstance, one could identify a quantity/distance function similar to that of panel (d) in every possible direction. As Figure 4-2 shows, by rotating the quantity/distance function about the vertical (quantity) axis, we circumscribe the seller’s market area for a given f.o.b. price. The distance from the seller’s location to the limit of market is called the market radius and is denoted by R in Figure 4-2.

This analysis leaves unanswered the question of how the monopolist chooses to establish a particular f.o.b. price. To address this issue, we must recall that a profit-maximizing firm will choose a price that is consistent with its setting marginal revenue equal to marginal cost. This decision criterion is common to spatial and nonspatial pricing analysis. However, the nature of demand, and therefore marginal revenue, is somewhat more complex in a spatial context.

Consider Figure 4-1 once more. If the monopolist seller were to set its f.o.b. price at p₀, we could measure the total quantity demanded at that price by the area under the quantity/distance function. That is, at every unit distance we can read the quantity demanded by the individual at that location by measuring the height of the quantity/distance function.

If there is one buyer at every unit distance, the total quantity demanded would be given by the summation of all the individual quantities.

When the buyers are evenly distributed in all directions over the area surrounding the seller, as represented in Figure 4-2, we have what is called a demand cone. Its height at any given distance from the seller’s location represents the quantity sold per buyer, and the volume of the cone represents the quantity demanded over the entire market area if the price p₀ is established at the seller’s location.

It is now possible to define the firm’s spatial demand curve. For each price, such as p₀, that is set at the seller’s location, a new demand cone will be established. A lower f.o.b. price implies a larger quantity demanded, for two reasons. First, because the nonspatial, individual demand curves are negatively inclined; when consumers are faced with lower prices they buy more. Second, the lower the f.o.b. price the larger the market radius, and hence the market area. Thus the number of buyers within the market area of the firm also depends on the f.o.b. price established. The spatial demand curve relates f.o.b. price to the quantity demanded over the entire market area, accounting for these two effects. Such a spatial demand curve is shown in Figure 4-3 and is labeled D_s.

Note that the spatial demand curve is convex to the origin. Its shape stems directly from the two effects mentioned above. Because the non-spatial demand curve is negatively inclined, we expect that higher (lower) prices will decrease (increase) the quantity demanded in a spatial context as well. However, because the market area, and therefore the number of customers, changes with each change in f.o.b. price, we should not expect the relationship between price and quantity demanded to be linear, even when there is a linear nonspatial demand curve and when the transfer cost gradient is linear.³ Recognizing the usual tendency of transfer costs and rates to increase less than proportionally with distance, we find still further basis for the usual convexity of the spatial demand curve.

Having established the nature of the spatial demand curve, it is now possible to extend our understanding of pricing decisions to a spatial context. Let MC in Figure 4-3 denote the locational unit’s marginal cost curve and MR_s denote its spatial marginal revenue curve. The profit-maximizing firm will equate MC and MR_s, and establish the f.o.b. price p_*. Once p _*is determined, a demand cone is also established, and its volume will be equal to q_*. Note also that the pricing decision results in establishing a market area for the unit. Thus if we are to understand the nature of market areas, we must also understand the motivations that guide pricing decisions.

This analysis permits us to enumerate some basic determinants of a locational unit’s market area when the presence of other sellers is not considered. When f.o.b. pricing is maintained, we must look to the nature of transfer costs and demand as well as to production costs in order to explain the existence of a market boundary. It is important to note, however, that if other pricing strategies are used, the nature of market boundaries may be affected.

With this background, we may go beyond consideration of a spatial monopolist in isolation and recognize that the effective area over which "monopoly power" can be exercised is often limited by the location of rival sellers. Thus market-area patterns emerge for various activities.

4.2.3 Market-Area Patterns

The simplest case of market-area patterns to consider is that involving a completely standardized output, equal operating costs for all sellers, and transfer costs increasing linearly with distance. The preceding analysis defined the natural market area of a seller as being limited by transfer costs. Potential buyers were confronted by a delivered price, and their decision to purchase or refrain from purchasing determined the area over which the monopolist had effective control. If the output in question is standardized and is offered for sale by more than one establishment, the customer’s choice is not simply one of whether to buy and how much; he or she must also decide which seller to patronize. To simplify matters we shall consider initially market-area patterns that result when all sellers of a standardized output have equal operating costs, face identical transfer costs that increase linearly with distance, and establish the same f.o.b. price.

Between any two sellers’ locations under these conditions, the market-area boundary will be a straight line that bisects at right angles a line drawn between the two locations. For all markets on one side of the line, the seller on that side has the advantage of lower output-transfer cost; on the other side of the boundary, the other seller has the advantage. In any direction where there is no competition, a seller’s market area will extend outward to some limiting distance beyond which there will be no sales at a price that would cover costs including transfer: That part of the market-area boundary, then, will be a circular arc. This situation is shown in Figure 4-4 for a set of four competing sellers.

The case just described is, of course, too simplified to represent any real situation; but it serves as a convenient point of departure for discovering the effects of various more realistic conditions upon market-area patterns. First, the costs at the two selling locations are unlikely in practice to be exactly equal. If they are unequal, the market-area boundaries look more like the one in Figure 4-5, bending away from the lower-cost seller’s location. The boundary, in this case, comprises all markets at which the sellers’ cost differential at their respective locations is exactly offset by the extra transfer cost from the lower-cost seller.⁴ Under our assumption of transfer costs rising linearly with distance, the boundary can never be a closed curve—that is, the lower-cost seller can never have a market area entirely surrounding that of the higher-cost seller.

Another possibility is that the two sellers incur different costs of transfer per ton per added mile. The result is shown in Figure 4-6 for a set of three sellers, with B’s transfer costs lower than those of A or C. This might reflect the situation if, for instance, firm B is shipping its product in a more easily transportable form, is conducting its own transport operation with superior efficiency, or has been able somehow to make more advantageous arrangements with transport contractors than have its competitors. The market-area boundary is now a closed curve: B’s market area completely surrounds those of A and C (the white areas). In this particular situation, we have the additional curious result that B cannot sell at its home location but only elsewhere!

Figure 4-7 demonstrates that market-area surrounding can occur even if both sellers are subject to the same transfer tariff—simply by virtue of the characteristic long-haul discounts.

Market-area surrounding of this type, resulting from the normally convex shape of transfer rate gradients, is extremely common in practice. Consider, for example, the circulation area of a major metropolitan newspaper in relation to the circulation areas of suburban and small-town newspapers in the same region, or the market areas of "national" brands of beer vis-à-vis those of local brands. The counterpart in terms of supply areas appears in small-city milksheds completely surrounded by the large milkshed of a larger city. The geographic price pattern for the product, in this case, is like that of a land surface rising to a mountain peak but punctuated with various hillocks and mounds on the slopes.

4.3 SOME ASPECTS OF SPATIAL PRICING POLICY AND MARKET AREAS

4.3.1 Market-Area Overlap

So far in this discussion of market and supply areas, we have concentrated on the development of market boundaries for fully standardized products. In each instance the seller’s market area comprises those markets that it can supply at a lower delivered cost (costs at the seller’s location plus transfer charges) than the sellers at any other locations. Under these circumstances, we might expect cleanly defined areas, similar to those mapped in Figures 4-4 through 4-7.

In practice, however, market-area and supply-area boundaries are blurred, and the areas overlap somewhat. This can result from absorption of part of the added transfer costs of distance by any of the three parties involved: the transfer agency, the buyers, or the sellers.

In the case shown in Figure 4-8, the transfer agency is the absorber. Reference was made earlier, in Chapter 3, to the fact that transfer rate schedules are sometimes simplified by setting a uniform rate over a whole "mileage block" or range of distances, if competitive conditions permit. When this is done, there are likely to be zones where the areas of two or more sellers overlap, as shown schematically in Figure 4-8. We must bear in mind, however, that the time taken in transfer is often important as well as the rate charged; and except in telecommunications and electric energy distribution, longer hauls take more time. Accordingly, not every case of rate bracketing results in market-area overlap.

The buyers can be regarded as absorbing some of the extra transfer costs of distance whenever they do not rigorously observe the principle of buying the cheapest good or service of a given type. Similarly, they can be regarded as absorbing some transfer costs if they are doing the transferring themselves (as in the case of retail shopping), but fail to patronize the most easily accessible seller. In the real world, the buyer does not often show this impartiality toward competing sellers, but for one reason or another has a preference even if the prices are equal. Such preference is least likely to be an important consideration in business purchases of such standardized goods as wheat or cement, and it is most likely to occur for retail purchases of such highly differentiated or even "personalized" items as medical or educational services, high-fashion clothing, and recreation. It is important to note that the buyer-preference factor will produce market-area overlap, but only to the extent that buyers have diverse preferences. Thus in Figure 4-9 (where it is assumed that A produces more cheaply than B), the line CC might be the market-area boundary for buyers who are indifferent to the relative qualities of A’s and B’s wares and would simply choose whichever is cheaper at their location. For those who believe that A’s product is really worth 5 cents a pound more than B’s, the boundary will be DD, which runs through points where the delivered cost from A is 5 cents greater than that from B. For those who believe that B’s is worth 5 cents a pound more than A’s, the boundary will be FE. Assuming that at every location there are buyers representing the whole intermediate gamut of preferences, the "boundary" or zone of overlap will comprise the belt between DD and FE. Both A and B will make sales throughout this overlap zone, though each will predominate in the part that is closer to him or her.

Finally, it may be sellers who are absorbing some of the added costs of distance. This is quite common. Indeed, the one case where this is not likely to happen is the special case mentioned earlier, in which the sellers are branch units of a single firm, public agency, or other multilocation decision unit. It is ordinarily in the interest of a firm or agency to distribute the product from its various facility locations in such a way as to minimize the total cost of supplying any given pattern of demand. This will ordinarily rule out cross-hauling or overlap of the market areas of those facility locations (except to the extent that it might reflect transfer cost absorption on the part of buyers or transfer agencies, as already considered). Accordingly, specific sales territories are allotted to the various branches. These market areas tend to be larger for branches with lower cost or higher capacity, and larger where demand is sparse than where it is dense.

Such definitive demarcation of areas is even more prevalent in public and administrative agencies. The Federal Reserve System divides the United States into twelve districts, and within some districts there are subdistricts such as that of the Pittsburgh branch of the Cleveland Federal Reserve Bank. Similarly, every federal government agency with field activities has its set of districts exclusively allocated to their respective branch office.⁵

In other activities, however (including most lines of business), the market rivalry between selling locations mainly involves rival firms, rather than different branches of the same firm. This situation introduces considerable possibilities for transfer cost absorption and consequently market-area overlap, depending on the pricing policies that the firms find advantageous.

4.3.2 Spatial Price Discrimination

Thus while we have assumed f.o.b. pricing in much of the preceding analysis, many other pricing policies can be established.⁶ If at any one location there is just a single seller or a small enough number to cooperate with one another, there are inviting opportunities to extend that location’s market area by "absorbing freight"—that is, discriminating in favor of more distant buyers. The extreme situation involves complete freight absorption, with the seller paying all transfer charges (but presumably setting a price that covers average delivery costs plus other costs). In that case, each seller sells at a uniform delivered price to buyers in various locations but receives a smaller net revenue per unit on its sales to the more distant buyers. Each seller then can afford to serve only those markets within a maximum distance determined by how much transfer cost it can afford to pay and still cover its out-of-pocket costs. Market areas will overlap if the sellers are sufficiently close together. In the zone of overlap, all the participating sellers share equally in sales. It is still to the interest of each seller (insofar as it is market-oriented) to locate close to concentrations of demand and far from competitors.

More sophisticated pricing policies entail a partial and selective absorption of transfer costs by the seller: Neither the f.o.b. price nor the delivered price is uniform on sales to different markets. The resulting patterns of prices and market areas will depend largely on the extent to which competitive pricing is based on short-term or long-term advantage.

The various sellers may take a long-term view of the possibilities and decide that they will all be better off the more closely they can collectively approximate the behavior of a single profit-maximizing monopolist. Such a monopolist, if it likewise took a long-term view, might well set its prices somewhat below levels that would yield the maximum immediate profit, in order to avoid encouraging the entry of new firms.

If the sellers do pursue such a policy of complete collusion, cooperation, or foresight (whichever term may be appropriate for the ease in hand), they will behave like branch units of a monopolistic firm or agency, which means that in general they will observe clean-cut market-area boundaries and avoid unnecessary transfer costs, such as might be involved in cross-hauling. There could still be market-area overlap, but only to the extent that the transfer agency or the buyers absorb transfer costs in the ways discussed earlier (involving mileage-block rates and qualitative preferences respectively).

How much of the transfer charges will be absorbed by the sellers assuming they are not under any external prohibition against spatial price discrimination? Presumably, the answer will be the same regardless of whether we consider an actual monopoly with separate branch locations or a set of sellers at different locations who find it in their mutual interest to price as would a single monopoly.

It turns out that (if we assume linear demand schedules at the markets) the sellers will maximize their profits by systematically discriminating against the nearer markets, absorbing exactly half of the transfer expenses.

(The remainder of this subsection may be skipped without loss of continuity.)

In order to appreciate this, consider the pricing decision depicted in Figure 4-10. The lines D_aand D_brepresent (nonspatial) demand curves associated with two buyers who have identical preferences and income but who reside at different distances from the seller’s location. We will assume that a buyer who is located adjacent to the seller (at distance 0) has the demand curve D_aand that the other buyer is located some distance away.

The demand curves in Figure 4-10 are drawn from the seller’s perspective, in that they show the relationship between the quantity demanded and the net price received by the seller—that is, delivered price less transfer costs. The vertical distance p₀, — p’₀between demand curves is a measure of the transfer costs between the two locations. The seller realizes that for any given quantity, the buyer represented by D_bwould be willing to pay a lower net price for the good in question because of the transfer costs associated with the buyer’s more distant location. Conversely, for the same f.o.b. price established by this seller the more distant buyer would be willing to purchase a smaller quantity. Thus distance affects demand, and this distinguishes otherwise identical buyers in the eyes of the seller.⁷

For simplicity, let the marginal costs of production be equal to zero.⁸ The marginal cost curve then coincides with the quantity axis. A monopolist equating marginal revenue and marginal cost in each market (that is, for each buyer) would establish an f.o.b. price of p’₁ for that which is adjacent to its location and a price of p’₁ for that which is more distant.

The difference in f.o.b. prices, p₁ — p’₁, is exactly one-half of the transfer cost to the more distant customer. For the proof of this statement refer to Figure 4-11, where the demand curve D_a has been reproduced. The marginal revenue curve (MR_a) associated with this demand curve bisects the quantity axis.⁹ Thus q₁ =(1 /2)q₀. Further, it is also the case that p₁=(1/2)p₀. The reason for this is that the triangles 0p₀q₀ and p₁p₀c are similar. Therefore, since p₁c =(1/2)0q₀, it follows that p₁p₀=(1/2)0p₀or, alternatively, p₁ =(l/2)p₀.

With this in mind, we may refer to Figure 4-10 and state that

p₁ —p’'₁ =(1/2)p₀ — (1/2) p’₀

=(1/2)( p₀ — p’₀).

Since p₀ — p’₀ is the transfer cost to the more distant location, we see that the monopolist has absorbed exactly one-half of these costs by setting a lower f.o.b. price for the more distant buyer.¹⁰

If the sellers’ locations and the market locations are given, the market-area boundaries will be in the same places regardless of whether the sellers follow this ideal discriminatory pricing policy or a nondiscriminatory policy under which delivered prices include the full transfer costs. Indeed, the areas will still be unchanged if the monopoly firm or the monopoly-simulating set of sellers chooses to absorb all of the transfer charges and sell at a flat delivered price, while at the same time choosing to avoid cross-hauling. This situation will, of course, require that the market-area boundaries as well as the uniform delivered price be agreed to and specified.

4.3.3 Pricing Policy and Spatial Competition

If the individual sellers are not so far-seeing or cooperative as we have here assumed, they will try to invade one another’s market areas by cutting prices. Consider the situation diagrammed in Figure 4-12, where sellers at A and B are competing for markets along the line between them, AB. The out-of-pocket costs of the two sellers at their own locations are AC and BD. Each, initially, is selling on the basis of an ideal system of price discrimination in favor of remote buyers and absorbing half the transfer costs; thus A’s delivered prices follow the gradient EF, and B’s follow the gradient GF. The lines CI and DH represent out-of-pocket costs plus full transfer costs from A and from B respectively. It should be noted that the ideal discriminatory delivered prices EF and GF rise at exactly half the slope of CI and DH, since the sellers are systematically absorbing half of the transfer costs. The market-area boundary is at L, where the delivered prices are both equal to FL.

In this situation, A may see a short-run gain in undercutting B’s delivered prices to points as far as M, thus stealing the market territory LM away from B. The possible invasion cannot go any farther, however, because when firm A sells to point M at a delivered price MI it is barely covering its out-of-pocket costs including transfer charges. Firm B can logically be expected to retaliate by cutting its delivered prices along the whole stretch KM, thus staging a counterinvasion of A’s market area. Carried to its logical conclusion, this game will produce a delivered price schedule EHJIG. Between K and M, A and B will be sharing the market. What will have happened is that the market-area boundary will now be a zone rather than a line; the sellers will both be making less profit; and the pattern of locational advantage for the buyers will have been changed, with locations in the competitive zone KM having now become more economical than they were before. The shaded area in the figure shows the maximum extent of price cutting.

The various cases discussed do not by any means exhaust either the theoretical possibilities or the variety of spatial pricing systems actually used by firms. Notably, there is the "basing-point" system, which has at various times been used in selling steel and other products. It is most often used in situations where the sellers are few and their market orientation is strongly constrained by access to transferable inputs, large-scale economies, and large fixed investments, and where the amount and location of demand fluctuate widely. In a basing-point system, a distinct pattern of delivered prices is observed: The price at any market is the lowest sum of the fixed f.o.b. price at a basing point plus the actual transfer charges from the basing point to the market. That is, sellers base their price on that charged at some other place, the basing point. For example, the place used as the basing point may be the largest supplying area for the commodity being sold. Thus unless government price regulation is in force, one might find that the price of crude oil in any U.S. city is based on the price established by the Organization of Petroleum Exporting Countries (OPEC) for crude oil from the Persian Gulf. In this case, an American producer who is shipping crude oil from Houston to Chicago might charge a price equivalent to the price of OPEC crude oil delivered to Chicago.

The economic incentive for a pricing system of this sort is easy to understand. If the producers in a given region (or country) cannot produce enough to satisfy local demand at the equilibrium price, local producers would be giving up profits if they charged any price lower than that of an identical commodity being imported by the region (country). The price of OPEC oil delivered to Chicago represents the maximum price that can be charged by the Houston producer. Unless there is competitive undercutting of price by other producers, the OPEC price can prevail.

In such a system, all sales entail either freight absorption or phantom freight charges, except those by a seller at a basing point to markets within the area governed by its basing point; there is likewise a considerable amount of market-area overlap and cross-hauling. For further discussion of this and still other variants, the curious reader will have to look elsewhere.¹¹

4.4 COMPETITION AND LOCATION DECISIONS

The preceding discussion of market areas and spatial pricing policies has described the behavior of sellers at given locations. We have recognized one important dimension of competition in a spatial context: the ability of locational units to absorb transfer costs. Thus spatial pricing policies serve as one mechanism by which firms may seek to gain competitive advantage. We now proceed by recognizing that the choice of location may itself be part of a competitive strategy.

In order to establish a simple framework for exposing the essential character of this aspect of spatial competition, we draw on a model developed by Harold Hotelling.¹² Our attention will be focused on two competitors who confront a linear-bounded market. It is assumed that production costs are zero for each locational unit. Identical buyers are evenly distributed over this market. Their demand for the good in question is not sensitive to price differences (the elasticity of demand is zero). One unit of the good is consumed by each individual per period of time, and each buyer prefers to purchase from the nearest seller.

This situation is depicted in Figure 4-13. In panel (a), the linear market, l, is segmented into two protected or uncontested parts, a and b, and one contested part, x + y, that is shared equally by the sellers. The two sellers, A and B, can move to any location on the line that will maximize their profit, and they do so believing that the rival will not change its location in response to their competitive action. We will assume that these moves are costless, in the sense that the sellers confront neither moving costs nor costs associated with disposing of fixed assets that might be associated with a given location.

In the restricted environment established by these assumptions, profits are always enhanced if a seller increases its market area. Since production is costless, larger market areas imply greater sales and, therefore, greater profits.

If each seller believed that the other’s location was fixed, the first seller to act, say A, would move to a position adjacent to its rival, ensuring itself the largest possible market area. If the initial positions are as depicted in panel (a), the first seller to move would seek to eliminate the contested portion of the market and maximize its protected portion. Thus panel (b) would represent such a move. The second seller is similarly motivated, however, and would leapfrog its rival to obtain competitive advantage. This type of movement would continue until neither seller stood to gain from further action. Such a situation would prevail if both sellers assumed central locations, each sharing one-half of the market.

These results demonstrate that some aspects of spatial competition may actually lead to the mutual attraction of sellers. In Chapter 5, other factors that might encourage clustering of this sort are examined in depth.

Some individuals have claimed great generality for Hotelling’s model, suggesting that it explains a good deal about spatial groupings of activity. This suggestion is difficult to justify, however, when one recognizes that attempts to move the model closer to reality by relaxing one or more assumptions have consequences that are very much at odds with Hotelling’s results. ¹³

The validity of this point is apparent if one explores the implications that follow when one assumes that the demand elasticity is non-zero and also allows for the possibility that sellers may act in light of a belief that rivals will react by competitive pricing or location decisions.

In earlier sections of this chapter, we have recognized that if the quantity demanded by individuals is sensitive to price, a seller that offers its goods for sale at a lower delivered price may be able to extend its market to include customers who are physically closer to competing establishments. Thus both price and location decisions can enter competitive strategy. In Hotelling’s model, not only was the demand elasticity equal to zero, but each seller’s expectation about the behavior of its competitor was naive; no change in the rival’s location was assumed. Now we wish to admit price responsiveness and somewhat more realistic expectations about competitive reactions in order to appreciate more fully the complexity of related problems.

While many possibilities might be examined that would serve to expose the character of decisions in this context, we choose to concentrate on two examples:¹⁴ (a) each seller assumes that any competitive price or location action that it takes will be matched by its rival, or (b) each seller assumes that its price changes will be met but that the rival’s location is fixed.

We continue to assume that there is a bounded linear market with uniformly distributed, identical buyers. Now, however, we also assume that they have negatively inclined linear demand functions. As with the Hotelling model, the sellers can move without cost and their marginal costs of production are zero; but we extend our assumptions concerning the sellers to include f.o.b. pricing with freight rates that are uniform over the market. The sellers are also profit maximizers.

Under these conditions, in situation (a), where each seller believes that price and location changes will be matched, neither seller can expect to gain from competitive behavior. Each believes that any attempt to lower the f.o.b. price in order to invade the rival’s market will be met and that the original boundary between the two sellers will be reestablished at that lower price. Similarly, each seller expects that any relocation aimed at invading the rival’s market will be matched and that the boundary separating the rivals will be maintained. Further, movements toward the rival inevitably imply movements away from buyers in the seller’s uncontested market segment. The associated increases in delivered price will affect demand.

There is pressure to avoid competition because of these circumstances. In fact, it has been suggested that a possible outcome in this situation would be for the sellers to cooperate and share the market equally, to their mutual advantage.¹⁵

In situation (b), price competition is eliminated. However, since each seller believes that the other’s location is fixed, both will move toward a central location. These moves are again at the cost of sales in the uncontested market segments as delivered prices rise for more distant consumers. Further, as in situation (a), there is no gain in the contested market segment. As both sellers approach the center, the interior boundary is unchanged.

Here, after their initial move toward the center, both sellers would realize that further movement in that direction would result only in additional losses. The tendency toward central locations has been checked as a result of competitive pressure and decreased sales to more distant customers. Thus we find that Hotelling’s results are very sensitive to assumptions concerning the nature of demand. Specifically, the elasticity of demand (which determines the extent of lost sales to the more distant customers) can be a factor in encouraging dispersed patterns of economic activity.

Once we admit possibilities of the sort just described, it is easy to recognize the complexity of the decisions faced by the firm. It must develop expectations about the behavior of competitors before choosing an initial location or deciding to relocate. Further, its pricing and location decisions are undertaken with the risk of retaliation. Any seller is likely to have little or no solid information on which to make the sort of judgments that are required.

Thus in addition to the substantial risks that may exist in any location or production decision because of uncertainty concerning market conditions, competition also implies uncertainty.¹⁶ The costs of guessing incorrectly may be substantial, and location decisions are undoubtedly influenced by this reality. In reacting to increases in uncertainty, firms will make more conservative production and location decisions: Their location choices, it has been suggested, are likely to reflect relatively smaller commitments of physical capital, and they will seek the security of locations with a variety of supply sources and good access to alternative markets).¹⁷

4.5 MARKET AREAS AND THE CHOICE OF LOCATIONS

4.5.1 The Location Pattern of a Transfer-Oriented Activity

In light of considerations thus far discussed, we can now formulate some general propositions about the locational preferences of a transfer-oriented activity.

Regardless of the price strategy involved, an output-oriented seller will still try to find the most rewarding location in terms of access to markets. It will not simply be comparing individual markets nor, as a rule, access to all markets wherever situated. Rather, it will have to evaluate the advantage of any location on the basis of how much demand there will be within the market area that it could expect to command from that location. Each location that it might choose entails a market area and a sales potential determined by where the buyers are and where the competition is.¹⁸

The best location from this viewpoint is one where demand for the seller’s kind of output is large relative to the nearby supply. This suggests that the seller will look for a deficit area, one into which the output in question is flowing, in preference to a surplus area, one out of which it is flowing. The direction of flow is "uphill," in the sense of an increasing price of the output; thus the seller will be attracted toward peaks in the pattern of prices, rather than toward low points. In other words, it will try to find the largest gap in the pattern of already established units of its activity as the most promising location for itself. If demand for the outputs of its activity were distributed evenly, the seller would simply look for the location farthest from any competition: that is, the center of the largest hole in the pattern. Since any new unit will aim to fill gaps in this way, the tendency will be toward an equal spacing of units of the activity, with market areas of approximately equal size and shape.

Analogously, input-oriented location units will look for surplus areas for that input; and if the supply curve for the input is the same over a large area, the units will tend to distribute themselves equidistantly, with supply areas identical.

In the real world, of course, no such regularity is found. Neither demand nor supply is spread evenly, competitors and sites are not identical, transfer costs are not the only factor of location, and transfer costs do not rise regularly with distance in all directions.

4.5.2 Transfer Orientation and the Patterns of Nonbusiness Activities

As was noted earlier, market areas and supply areas are not peculiar to profit-motivated activities. Public agencies, and a variety of private and semipublic institutions whose outputs and inputs are mainly services given rather than sold, are likewise subject to the factors of transfer cost and scale economy that give rise to market-area or supply-area patterns. In some cases, the boundaries of such service areas are administratively defined and perfectly clean-cut; for example, police or electoral precincts, dioceses, tax collection districts, areas of citizens’ associations, or chapter areas of a fraternal lodge or professional association. In others, there is a considerable market-area overlap. Thus church worshipers or communicants need not choose the nearest church of their denomination; and colleges, welfare agencies, and social clubs likewise compete spatially, though generally they have limited areas of market dominance. There are always added transfer costs in operating at a greater distance, but these can be absorbed by the provider, the transfer agency, or the recipient of the service.

The principle of mutual repulsion among units of the same transfer-oriented activity likewise holds good in many nonbusiness activities. Thus a philanthropic agency, group, or individual setting up neighborhood recreation centers or nursery schools in an urban ghetto will be able to give better service if the units are spaced so that they are more accessible from different parts of the "market," and each will have its "market area."

Still further extension of the concept of attractive and repulsive forces is involved when we recognize such factors as the individual’s desire for privacy. Human beings and other animals have strong preferences for maintaining certain critical distances from their fellows, when interacting socially or even when simply minding their own business, and social anthropologists have uncovered some interesting ethnic and intercultural differences as to what is regarded as the optimum degree of proximity. The study of these preferences and their physical and psychological bases has obviously much to contribute to our understanding of the stresses induced by crowding and to the proper design of facilities for urban living—here as elsewhere, the economist becomes keenly aware of the limitations of a narrow disciplinary approach in dealing with complex human problems.¹⁹

4.6 SUMMARY

The location pattern of an industry or other "activity" changes partly as the result of deliberate moves or choice of new locations, but also as the result of the competitive survival and growth of well-located units and the disappearance or shrinkage of badly located ones.

In some activities, the principal locational interaction among the units is mutual repulsion—each seeks to keep its distance from others. This is generally the case when the activity is market-oriented and the market is dispersed, or when the activity is input-oriented and the sources of input are dispersed. In the former case, each unit has its own market area; in the latter, each has its own supply area. In general, statements about market areas of sellers can be applied, mutatis mutandis, to supply areas of buyers.

The concept of demand in a spatial context is somewhat more complicated than that associated with nonspatial analysis. The process by which firms make price and output decisions reflects the fact that customers are distributed over space.

The market-area boundary between two sellers of the same good, with equal production and input costs, is a straight line midway between the sellers. If one seller has a cost advantage, the boundary will be farther from it and concave toward its higher-cost competitor. If sellers do not pay the same transfer rates per mile, or if transfer rates are less than proportional to distance (as is quite usual), the higher-cost sellers can have their market areas completely surrounded by those of lower-cost sellers. Market-area overlap is common and can reflect absorption of transfer costs in the overlap zone by sellers, buyers, or the transfer agency.

The complex nature of competitive spatial pricing and location decisions is a source of substantial uncertainty to firms. They must be concerned with the actions and reactions of rivals. Some competitive pressures may actually draw sellers toward more central locations, but the potential loss of sales to customers in outlying areas serves, at least partially, to offset this tendency.

TECHNICAL TERMS INTRODUCED IN THIS CHAPTER

SELECTED READINGS

Brian J. L. Berry, Geography of Market Centers and Retail Distribution (Englewood Cliffs, N.J.: Prentice-Hall, 1967).

Melvin L. Greenhut, Microeconomics and the Space Economy (Chicago: Scott, Foresman, 1963).

M. L. Greenhut and H. Ohta, Theory of Spatial Pricing and Market Areas (Durham, N.C.: Duke University Press, 1975), Chapters 1-6.

David D. Haddock, "Basing-Point Pricing: Competitive vs. Collusive Theories," American Economic Review, 72, 3 (June 1982), 289-306.

Harry W. Richardson, Regional Economics (Urbana: University of Illinois Press, 1978), Chapters 2-3.

Daniel F. Spulber, "Spatial Nonlinear Pricing," American Economic Review, 71, (December 1981), 923-933.

Charles M. Tiebout, "Location Theory, Empirical Evidence, and Economic Evolution," Papers and Proceedings of the Regional Science Association, 3 (1957), 74-86.

Michael J. Webber, Impact of Uncertainty on Location (Cambridge, Mass.: MIT Press, 1972), Chapters 5-8.

APPENDIX 4-1

Conditions Determining the Existence and Size of Market Areas

Among the spatial pricing policies that may be adopted, several have been given special attention in the literature concerning this topic. These include the establishment of (1) a uniform f.o.b. price, (2) a uniform delivered price, and (3) selective price discrimination.²⁰ These policies are directly related to the amount of transfer costs that a seller chooses to pass along to customers. Thus f.o.b. pricing is defined as a situation where each customer pays the full cost of transfer to his or her location, whereas under uniform pricing a single price is charged to all customers regardless of their location, and in effect some customers pay more than the actual transfer costs to their location while others pay less. In Section 4.3.2 it was demonstrated that with linear demand curves, optimum discrimination would require that one-half of the transfer charges be passed along and one-half be absorbed by the seller.

The pricing policy will have important effects on the size of the seller’s market area and the seller’s profits. It will even determine the conditions under which sales from a particular location are viable, in the sense that they are consistent with the seller realizing normal profits. In order to demonstrate these points, we shall make use of some theoretical results obtained by Martin Beckmann concerning the pricing decision of a spatial monopolist.²¹

The following analysis applies to a highly simplified situation. Demand for the seller’s product is uniform over the whole area, sales per unit of area being g (h — m) where m is the delivered price and h is the price above which no one will buy; g reflects the "market density." Transport costs are uniformly t per unit quantity and distance. The total costs of production are given by f + qc, where f is fixed cost, c is unit variable cost (=marginal cost), and q is volume of output. To simplify the analysis still further, market areas are treated as if they were circular in all cases. Distance of a buyer from the selling center is denoted by r, and the market area radius by R.

Under these conditions, Beckmann²² has shown that a monopolistic seller can maximize its profits by setting prices as follows:

*In the f.o.b. and optimum-discrimination cases, delivered price rises with increased distance from the market and at the edge of the market is equal to h (the price at which buyers stop buying). It is assumed that in the case of flat delivered price, the seller will refuse to sell to buyers beyond the trading-area boundary: Though they would be willing to buy, the seller could not cover its variable cost and transfer cost on such sales.

It will be observed that the optimum radius is greatest with 50 percent freight absorption, and is three-quarters that size (that is, the area is 9/16 as large) under either zero or 100 percent freight absorption.

The maximum profits attainable by the monopolist are:

1. With uniform f.o.b. price:

₀ò^R 2prg(p — c) (h — p — rt)dr — f

where p =f.o.b. price. This reduces to

(9pg/256) [(h — c)⁴/t²] — f=.110g[h — c)⁴/t²] — f

2. With uniform delivered price:

₀ò^R 2pfg(m — c— rt)(h — m)dr — f

where m =delivered price. This reduces to

(9pg/256) [(h — c)⁴/t²] —f=.110g[(h — c)⁴!t²] — f

the same as in the case of uniform f.o.b. price.

3. With optimum discrimination:

₀ò^R 2prg[(h — c — tr) /2]²dr — f

This reduces to

(pg/24) [(h — c)⁴ It²] — f=.131g[(h — c)⁴/t²] — f

It appears, then, that the returns applicable to fixed costs (that is, profits + f) for any given set of cost and demand conditions will be about 131 / 110=1.19 times as large under optimum discrimination as under either uniform f.o.b. or uniform delivered pricing.

The threshold conditions that have to be met in order for any seller to establish a trading area are shown by setting maximum profits at zero. These conditions are as shown below:

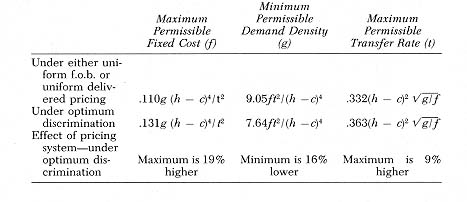
It is clear from these results that the chances for the existence of trading areas are favored by (1) lower fixed costs, (2) higher market density, (3) cheaper transfer, and (4) the exercise of rational price discrimination.

The size of trade areas, once they exist, is another question. The first table in this appendix shows that, for a monopolist, the most profitable trade area will be larger when transfer is cheaper (R is inversely related to t) and is independent of both fixed costs and demand density. When there is competition among sellers, trading areas will be larger if fixed costs (f) are greater or if demand density (g) is lower, but depend in a more complex way upon the levels of t, h, and c and the kind of pricing system the competitors use.

ENDNOTES

1. Martin Beckmann, Location Theory (New York: Random House, 1968), adopts a different terminology, in which "activity" corresponds to what we have been calling ‘location unit," and "industry" to what we call "activity."

2. E. M. Hoover, The Location of Economic Activity (New York: McGraw-Hill, 1948), p. 10. This point is further developed in Armen A. Alchian, "Uncertainty, Evolution, and Economic Theory," Journal of Political Economy, 58 (June 1950), 211-221; and in Charles M. Tiebout, "Location Theory, Empirical Evidence, and Economic Evolution," Papers and Proceedings of the Regional Science Association, 3 (1957), 74-86. Tiebout (p. 85) cites the case of brewing, in which "in the evolutionary struggle to survive, Milwaukee gained the dominant position," and that of the automobile industry, in which Detroit emerged as chief victor in the struggle. In both instances, personal or other "fortuitous" factors played a large part in the initial locations.

Another interesting case is that of the Hershey Chocolate Company, an early giant in its industry. Milton Hershey, having made candy successively but not very successfully in Philadelphia, Chicago, Denver, New York, and Lancaster, Pa., finally chose a rural Pennsylvania Dutch location for his famous factory and planned town of Hershey—largely because that was his birthplace. A rural location for a large candy factory was then almost unheard-of, and few expected him to survive. But the location happened to be an excellent choice in terms of access to milk and imported cocoa beans, nearness to the largest centers, and labor supply. Without those economic advantages, Hershey would probably have failed again. Joseph R. Snavely, Milton S. Hershey, Builder (Hershey, Pa: privately printed, 1935)

3. It can be shown that the spatial demand curve will be convex to the origin (concave from above) regardless of the shape of the nonspatial demand curve. See M. L. Greenhut and H. Ohta, Theory of Spatial Pricing and Market Areas (Durham, NC.: Duke University Press, 1975), pp. 19-20.

4. If transfer costs rise linearly with distance, and if seller A’s costs are $1 a ton lower than seller B’s, the distance of any point on the boundary from A will exceed the distance of that point from B by a fixed amount—the distance for which the line-haul cost of transfer is $1 a ton. The shape of the market-area boundary will be a hyperbola, since a hyperbola can be defined as the locus of all points whose distances to two fixed points differ by a fixed amount.

5. See also map Figure 9-3 and accompanying discussion.

6. The seller’s choice among spatial pricing policies may affect the size of the market area, profits, and even the feasibility of carving out a market area. See Appendix 4-1 for a discussion of the relationship between pricing policies, profitability, and the existence and size of market areas.

7. Note that the more distant buyer has a greater elasticity of demand at any f.o.b. price established by the seller. This follows from the fact that the elasticity of demand is defined as —[(dq/dp)(p/q)j. Since the slope, (dq/dp), is constant over the entire length of each demand curve and is the same for both demand curves, the fact that the more distant buyer would be willing to purchase a smaller quantity at any given f.o.b. price means that his or her demand curve is more elastic. Thus the feature that distinguishes these buyers, from the seller’s perspective, is this difference in their demand elasticity.

8. This assumption makes the graphical presentation to follow considerably easier and does not alter the conclusion. That this is true can be seen from the mathematical statement offered in footnote 10.

9. For any linear demand curve, the associated marginal revenue curve is exactly twice as steep and, therefore, bisects the line bounded by the origin and the quantity intercept. See Richard G. Lipsey and Peter 0. Steiner, Economics, 6th ed. (New York: Harper & Row, 1981), pp. 242-243.

10. This conclusion can also be reached algebraically as follows: Assume that at any market the sales are a — bp, where p is the delivered price, and that variable costs per unit of sales are c. Net receipts from sales to any market, over and above transfer expenses and variable costs, are then (a — bp) (p — c — t), where t is the unit transfer expense to that market. By differentiating this expression with respect to p and setting the derivative to zero, we find that the net receipts are maximized if p, the delivered price, is equal to [(c + a/b)/2] + t/2. The first term in this expression is the price that buyers are to be charged at the seller’s location, where transfer costs are zero. It is the average between c (variable Costs) and a/b (the price at which no sales would be made, that is, the vertical intercept of the demand curve). For sales to all other markets, the ideal delivered price increases with distance just half as fast as the transfer cost does. (Compare Appendix 3-1.)

11. For a discussion of several issues concerning basing-point pricing and additional references to this topic, see David D. Haddock, "Basing-Point Pricing: Competitive vs. Collusive Theories," American Economic Review, 72, 3 (June 1982), 289-306. Haddock points out that the basing-point system need not imply collusion among sellers, and he discusses the economic incentive for this pricing behavior when commodities are traded interregionally.

Handy references on the varieties of spatial competition and pricing systems include Beckmann, Location Theory, pp. 30-50; and M. L. Greenhut, Microeconomics and the Space Economy (Chicago: Scott, Foresman, 1963). Mathematical statements generalizing the theory of spatial pricing can be found in Martin J. Beckmann, "Spatial Price Policies Revisited," Bell Journal of Economics, 7, 2 (Autumn 1976), 619-630; and in Daniel F. Spulber, "Spatial Nonlinear Pricing," American Economic Review, 71, 5 (December 1981), 923-933.

12. Harold Hotelling, "Stability in Competition," Economic Journal, 39 (March 1929), 41-57.

13. B. Curtis Eaton and Richard Lipsey make this point in the development of their work. See Eaton and Lipsey, "Comparison Shopping and the Clustering of Homogeneous Firms," Journal of Regional Science, 19, 4 (November 1979), 421-435.

14. The framework for the analysis of the examples to follow was established by Arthur Smithies, "Optimal Location in Spatial Competition," Journal of Political Economy, 49 (June 1941), 423-439. Edward C. Prescott and Michael Vischer, "Sequential Location Among Firms with Foresight," Bell Journal of Economics, 8,2 (Autumn 1977), 378-393, substantially expand the theoretical perspective on related problems by examining the behavior of firms that try to anticipate the decision rules used by later entrants to the market.

15. The profit of each of the sellers would be maximized if they assumed quartile locations— that is, if the boundary between the sellers were at the midpoint of the market, and each seller located in the center of its market segment. In this way, average transfer costs on delivery of the product to customers in each market segment would be minimized, and sales would therefore be maximized.

16. We distinguish here between uncertainty concerning such factors as shifting markets, shifting sources of supply, transportation costs, taxes, etc. (or uncertainty concerning the "state of nature") as introduced in Chapter 2 on the one hand, and uncertainty concerning rivals on the other.

17. See Michael J. Webber, Impact of Uncertainty on Location (Cambridge, Mass.: MIT Press, 1972). These are but two examples of the implications that can be drawn from an analysis of location decisions under uncertainty. The interested reader will find Webber’s text a useful introduction to the related literature.

18. In an activity characterized by market-area boundaries that are blurred for any of the reasons discussed earlier, evaluation of the market potentialities of any location is somewhat more complicated: The locator must estimate what its market share will be in the penumbra of its market area where this overlaps with that of one or more competitors. See also the discussion in Section 2.8.

19. A fascinating popular treatment of such space relations as the anthropologist sees them is Edward T. Hall, The Hidden Dimension (New York: Anchor Books, 1966).

20. As noted earlier in this chapter, other spatial pricing alternatives are available. See, for example, the discussion on basing-point pricing and Daniel F. Spulber, "Spatial Nonlinear Pricing," American Economic Review, 71, 5 (December 1981), 923-933.

21. Beckmann, Location Theory.

22. lbid., pp. 32, 51, 52. Beckmann’s formulas have been translated here into our notation. He assumed t =l, and he wrote a/b where we have h, and b where we have g.